Related Topics
Healthcare Reform:Saving For a Rainy Day
Lifetime Health Savings Accounts
Fun With Numbers
The principles of compound interest are thought to have been a product of Aristotle's mind. The principles of passive investing are more recent, mainly attributed to John Bogle of Vanguard, although Burton Malkiel of Princeton has a strong claim. In the present section, we propose to merge the two methodologies, compound interest with passive investing, trying to give the reader some idea why the combination could supply Health Savings Accounts with seriously augmented revenue. Because there is so much political flux, it cannot be an actual plan until the politically-controlled numbers have some finality to them.
The proposal to accumulate funds, however, shifts responsibility to the customer to spend wisely, even resorting to employing some of the individual's taxable money to pay small medical costs, thus preserving the tax shelter. (Or to use escrow accounts, or over-deposit in some other way, such as reducing final goals.) HSA doesn't directly reduce health costs, it eliminates some unnecessary ones but provides lots of extra money to pay for essential ones. At the outset we want to state, schemes of this sort have a history of working effectively up to a certain level, and then begin to interfere with themselves as eager money rushes in. There's no sign of that so far, but it might appear. Therefore, we advise modest hedged experiments rather than attempts to pay for all of healthcare, reducing health costs perhaps by only a quarter or a half, since those smaller levels would still amount to large returns. Balancing the risks with investments outside the HSA -- is just another prudent way of hedging the bet.
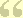 Money earning seven percent will double in ten years. 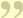
|
In fact, we have a tragic example in the nation's pension funds. A few decades ago, pension managers were tempted to invest in stocks rather than bonds, and then the stock market crashed, stranding pensioners with low rates of return, rather than the high ones they had hoped for. I want readers to understand I am well aware of the cyclicity of markets, and make these suggestions, regardless. As long as we include a thirty-year "Black swan" contingency by limiting coverage to a quarter or a third, it should be reasonably safe, but savings would still be enormous. There are other, more traditional ways of protecting endowments from stock crashes. With people of every age to consider, the long transition period alone would almost automatically buffer out black swans.
Having issued a warning to be a conservative investor, let's now introduce some notes of reassurance. Younger people are always likely to be healthier. Those who save their money while young therefore need not use all of it for healthcare -- for several decades. Compound interest works to magnify savings, the longer its horizon the better. We'll describe passive investing later, but it too should increase the average rate of return. These investments after some successes increase the incentives to save. If no one buys Health Savings Accounts, the incentives were apparently not large enough. If everyone rushes to buy, perhaps the incentives were unwisely too attractive. Right now, the financial industry is observing a rush to passive investing; nearly fifty percent of mutual fund investors are switching to "index funds" in spite of capital gains taxes on selling other holdings. Since the marvel of compound interest has been accepted for thousands of years, a mixture of compound interest and passive investing isn't an especially radical idea.
What's radical is the idea that all those highly-paid advisors can't do better than random coin-flippers. What's radical is to discover that the main ingredient of poor performance is high middle-man fees. Low fees won't assure high returns, but high fees will assuredly lead to low returns. If that new idea gets replaced in turn, it will be replaced by something better, and everyone should switch to it. But if compound interest is here to stay, this proposal is safer than it sounds. The investment income rate or continued employment of your agent is what isn't guaranteed, which is why business relationships (between customers and managers of HSAs ought to remain portable and transparent by law. Your manager might move, or you might decide to move away, from him.
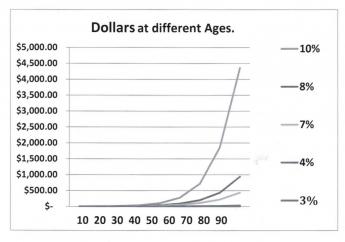
Start by looking at what happens if you jump your interest rate curve from 5% to 12%, or if you lengthen life expectancy from age 65 to age 93. That's what the graph is intended to show, and we stretch the limits to see what stress will do. Jumping to the highest rate (12%)the interest rate gets the balance to a couple of million dollars pretty quickly and lengthening the time period further enhances that gain. The combination of the two easily escalates the investment far above twenty million. The combination of extra time and extra interest rate thus holds the promise of quite easily paying for a lengthening lifetime of medical care, regardless of inflation. In fact, it gets the calculation to giddy amounts so quickly it creates suspicion.
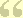 Average lifetime health costs: $350,000 per lifetime 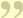
|
One person who does have practical control of the interest rate an investor receives is his own broker. The broker shares the income, but usually takes the first cut of it, himself. Covering a full century, Roger Ibbotson has published the returns on various investments, and they don't vary a great deal. Common stock produces a return of between 10% and 12.7% in spite of wars and depressions; if you stand back a few feet, the graph is pretty close to a straight line. You wouldn't guess it was that high, would you? If you don't analyze carefully, a number of brokerages offer Health Savings Accounts which produce no interest at all -- to the investor -- for the first ten years. Indeed, the income of 2% also amounts to nothing at all during a 2% inflation. In ten years, 2% approaches a haircut of nearly 20%, explained by the small size of the accounts, and by the fact that customers who know better will generally just politely look for another vendor. Since the number of accounts has quickly grown to be more than fifteen million, it might be time for some sort of consumer protection. The prospective future size of these accounts should command greater market power, quite soon. After all, passive investment should mainly involve the purchase of blocks of index funds, all with fees of less than a tenth of a percent . Much of this haircutting is explained by the uncertainties of introducing the Affordable Care Act during a recession and taking six years just to get to the point of a Supreme Court Test, to see if its regulations are legal and workable. It can be used to provide high-deductible coverage, but it's expensive.
That's the Theory. The rest of this section is devoted to rearranging healthcare payments in ways which could -- regardless of rough predictions -- easily outdistance guesses about future health costs. When the mind-boggling effects are verified, skeptics are invited to cut them in half, or three quarters, and yet achieve a worthwhile result. The purpose here is not to construct a formula, but to demonstrate the power of an idea. Like all such proposals, this one has the power to turn us into children, playing with matches. By the way, borrowing money to pay bills will conversely only make the burden worse, as we experience with the current "Pay as you go" method. By reversing the borrowing approach we double the improvement from investment, in the sense we stop doing it one way and also start doing the other. In the days when health insurance started, there was no other way possible. The reversal of this system has only recently become plausible, because life expectancy has recently increased so much, and passive investing has put that innovation within most people's reach. The environment has indeed changed, but don't take matters further than the new situation warrants.
Average life expectancy is now 83 years, was 47 in the year 1900; it would not be surprising if life expectancy reached 93 in another 93 years. The main uncertainty lies in our individual future attainment of average life expectancy, which we don't know, but probably could guess with a 10% error. When the future is thus so uncertain, we can display several examples at different levels, in order to keep reminding the reader that precision is neither possible nor necessary, in order to reach many safe conclusions about the average future. Except for one unusual thing: this particular trick is likely to get even better in the future. Even so, it is best to do only conservative things with a radical idea.
Reduced to essentials for this purpose, today's average newborn is going to have 9.3 opportunities to double his money at seven percent return and would have 13.3 doublings at ten percent. Notice the double-bump: as the interest rate increases, it doubles more often, as well as enjoying a higher rate. If you care, that's essentially why compound interest grows so unexpectedly fast. This widening will account for some very surprising results, and it largely creeps up on us, unawares. Because we don't know the precise longevity ahead, and we don't know the interest rate achievable, there is a widening variance between any two estimates. So wide, in fact, it is pointless to achieve precision. Whatever it is, it will be a lot.
|
| One Dollar: Lifetime Compound Interest |
Start with a newborn, and give him a dollar. At age 93, he should end up with between $200 (@7%) and $10,000 (@10%), entirely dependent on the interest rate. That's a big swing. What it suggests is we should work very hard to raise that interest rate, even just a little bit, no matter how we intend to use the money when we are 93, to pay off accumulated lifetime healthcare debts. Don't let anyone tell you it doesn't matter whether interest rates are 7% or 12.7%, because it matters a lot. And by the way, don't kid yourself that a credit card charge doesn't matter if it is 12% or 6%. Call it greed if that pleases you; these "small" differences are profoundly important.
If that lesson has been absorbed, here's another:
In the last fifty or so years, American life expectancy has increased by thirty years. That's enough extra time for three extra doublings at seven percent, right? So, 2,4,8. Whatever amount of money the average person would have had when he died in 1900, is now expected to be eight times as much when he now dies thirty years later in life. And even if he loses half of it in some stock market crash, he will still retain four times as much as he formerly would have had at the earlier death date. The reason increased longevity might rescue us from our own improvidence is the doubling rate starts soaring upward at about the time it gets extended by improved longevity. In particular, look at the family of curves. Its yield turns sharply upward for interest rates between 5% and 10%, and every extra tenth of a percent boosts it appreciably.
Now, hear this. In the past century, inflation has averaged 3%, and small-capitalization common stock averaged 12.7%, give or take 3%, or one standard deviation (One standard deviation includes 2/3 of all the variation in a year.) Some people advocate continuing with 3% inflation, many do not. The bottom line: many things have changed, in health, in longevity, and in stock market transaction costs. Those things may have seemed to change very little, but with the simple multipliers we have pointed out, conclusions become appreciably magnified. Meanwhile, the Federal Reserve Chairman says she is targeting an annual inflation rate of 2% of the money in circulation; the actual increase in the past century was 3%. If you do nothing at 3%, your money will be all gone in thirty-three years. If you stay in cash at 2%, it will take fifty years to be all gone.
But if you work at things just a little, you can take advantage of the progressive widening of two curves: three percent for inflation stays pretty flat, but seven percent for investment income starts to soar. Up to 7%, there is a reasonable choice between stocks and bonds; but if you need more than 7% you must invest in stocks. Future inflation and future stock returns may remain at 3 and 7, forever, or they may get tinkered with. But the 3% and 7% curves are getting further apart with every year of increasing longevity. Some people will get lucky or take inordinate risks, and for them, the 10% investment curve might widen from a 3% inflation curve, a whole lot faster. But every single tenth of a percent net improvement will cast a long shadow.
But never, ever forget the reverse: a 7% investment rate will grow vastly faster than 4% will, but if people allow this windfall to be taxed or swindled, the proposal you are reading will fall far short of its promise. Our economy operates between a relatively flat 3% and a sharply rising 4-5%. In other words, it wouldn't have to rise much above 3% inflation rate to be starting to spiral out of control. Our Federal Reserve is well aware of this, the public less so. A sudden international economic tidal wave could easily push inflation out of control, in our country just as much as Greece or Portugal. On the other hand, as developing nations grow more prosperous, our Federal Reserve will control a progressively smaller proportion of international currency. Therefore, we would be able to do less to stem a crisis that we have done in the past.
To summarize, on the revenue side of the ledger, we note the arithmetic that a single deposit of about $55 in a Health Savings Account in 1923 might have grown to about $350,000 by today, in the year 2015, because the stock market did achieve more than 10% return. There is considerable attractiveness to the alternative of extending HSA limits down to the age of birth, and up to the date of death. It's really up to Congress to do it. If the past century's market had grown at merely 6.5% instead of 10%, the $55 would now only be $18,000, so we would already be past the tipping point on rates. In plain language, by using a 10% example, $55 could have reached the sum now presently thought by statisticians -- to be the total health expenditure for a lifetime. By achieving a 6.5% return, however, the same investment would have fallen short of enough money for the purpose. Like the municipalities that gambled on their pension fund returns, that sort of trap must be avoided. Things are not entirely hopeless, because 6.5% would remain adequate if our hypothetical newborn had started with $100, still within a conceivable range for subsidies. But the point to be made provides only a razor-thin margin between buying a Rolls Royce, and buying a motorbike. If you get it right on interest rates and longevity, the cost of the purchase is relatively insignificant. That's the central point of the first two graphs. For some people, it would inevitably lead to investing nothing at all, for personal reasons. Some of the poor will have to be subsidized, some of the timid will have to be prodded. This is more of a research problem than you would guess: a round-about approach is to eliminate the diseases which cost so much, choosing between different paths of research to do it, or rationing to do it. Right now we have a choice; if we delay, the only remaining choice would be rationing.
Commentary.This discussion is, again, mainly to show the reader the enormous power and complexity of compound interest, which most people under-appreciate, as well as the additional power added by extending life expectancy by thirty years this century, and the surprising boost of passive investment income toward 10% by financial transaction technology. Many conclusions can be drawn, including possibly the conclusion that this proposal leaves too narrow a margin of safety to pay for everything. The conclusion I prefer to reach is that this structure is almost good enough, but requires some additional innovation to be safe enough. That line of reasoning will be pursued in a later chapter.Escrow Accounts and Over-Depositing. The main unpredictable feature of these future projections is you can't predict when you will get sick and deplete the account. Money withdrawn early is much more damaging than money withdrawn late in the cycle. Catastrophic insurance will somewhat protect against this risk, but the safest approach is to use segregated, somewhat untouchable, escrow accounts for future heavy expenses. That, combined with deliberate over-depositing, is the safest approach. If Obamacare would settle down, it might serve that function, as well, but the political situation is pretty unsettled until a large-group design is made final, and that seems to mean November 2016 at the earliest.Revenue growing at 10% will rapidly grow faster than expenses at 3%. As experience has shown, it is next to impossible to switch health care to the public sector and still expect investment returns at private sector levels. Repayment of overseas debt does not affect actual domestic health expenditures, but it indirectly affects the value of the dollar, greatly. Without all its recognized weaknesses, a fairly safe description of present data would be that enormous savings in the healthcare system are possible, but only to the degree, we contain next century's medical cost inflation closer to 2% than to 10%. The simplest way to retain revenue at 10% growth, on the other hand, is by anchoring the price to leading healthcare costs within the private sector. The hardest way to do it would be to try to achieve private sector profits, inside the public sector. This chapter describes a middle way. It's better than alternatives, perhaps, but not miraculous.
Cost, One of Two Basic Numbers. Blue Cross of Michigan and two federal agencies put their own data through a formula which created a hypothetical average subscriber's cost for a lifetime at today's prices. The agencies produced a lifetime cost estimate of around $300,000. That's not what we actually spent because so much of medical care has changed, but at such a steady rate that it justifies the assumption, it will continue into the next century. So, although the calculation comes closer to approximating the next century (than what was seen in the last century) it really provides no miraculous method to anticipate future changes in diseases or longevity, either. Inflation and investment returns are assumed to be level, and longevity is assumed to level off. So be warned. This Classical HSA proposal, particularly with merely an annual horizon, proposes a method to pay for a lot of otherwise unfunded medical care. The proposal to pay for all of it began to arise when its full revenue potential began to emerge, rather than the other way around. If a more ambitious Lifetime HSA proposal ever works in full, it has a better chance, but must expect decades of transition before it can. Perhaps that's just as well, considering the recent examples we have of being in too big a hurry. Rather surprisingly, the remaining problem appears merely a matter of 10-15% of revenue, but all such projection is fraught with uncertainty.
Revenue, The Other Problem. The foregoing describes where we got our number for future lifetime medical costs; someone else did it. Our other number is $150,750, which is our figure for average lifetime deposit in an HSA. It's the current limit ($3350 per year of working life) which the Congress applied to deposits in Health Savings Accounts. No doubt, the number was envisioned as the absolute limit of what the average person could afford, and as such seems entirely plausible. You'd have to be rich to afford more than that, and if you weren't rich, you would certainly struggle to afford so much. To summarize the process, the number amounts to a guess at what we can afford. If it turns out we can't afford it, this proposal must be supplemented, and the easiest expedient is to raise the contribution limits. Other alternatives are pretty drastic: to jettison one or two major expenses, like the repayment of our foreign debts for past deficits in healthcare entitlements, or the privatization of Medicare. Not privatizing Medicare sounds fine to most folks, but they probably haven't projected its coming deficits. It would leave us considerably short of paying for lifetime health costs for quite a long transition period, but it might be more politically palatable, like Greece leaving the Euro, than paying more. Almost anything seems better than sacrificing medical care quality, which to me is an unthinkable alternative, just when we were coming within sight of eliminating the diseases which require so much of it.
Originally published: Monday, September 29, 2014; most-recently modified: Sunday, July 21, 2019